SESSION 1

PLASMA TORCHES

INTRODUCTION

Electrical arcs are used in a multitude of industrial applications: arc lamps for lighting, arc welding for joining together pieces of metal, arc furnaces for the melting of steel, arc torches for the coating of materials and in circuit breakers for the interruption of electric currents. Arcs also occur in nature in the form of lightning, one of the most spectacular phenomena of the natural world.

There have been many efforts to model electric arcs, particularly recently, when with the availability of high speed computers we have the capacity to make calculations and predictions of a complexity that has never before been possible. If we can model the principle properties of an arc whose properties we know, we have the capacity to understand the basic physics and processes of the arc. What we would really like to do is have a modeling capacity to be able to make predictions of arc properties for which we have not yet done experiments. For example, to predict the luminous efficiency of a new lamp using a plasma from a new gas mixture, or whether a circuit breaker of new design will interrupt a given current from a particular circuit, or what will be the heat affected zone in welding a particular device made from a different type of metal. In these complex problems, arc modelers have had limited success. But our capabilities are improving rapidly.

BASIC PHYSICAL PROCESSES

In the various attempts to model arcs, significant physics problems have been encountered in every region of the arc.
(a) The arc column: Generally it is assumed that the plasma in the arc column is in local thermodynamic equilibrium. That is, we assume that the temperature of all constituents of the plasma, namely electrons, neutral particles and ions, are all the same at any given point. Then we can obtain by the theory of chemical equilibrium, the composition of the plasma for any gas mixture, temperature and pressure. Then it is possible to calculate the electrical and thermal conductivity and also the viscosity of the plasma at this temperature and pressure. But it has to be mentioned that this basic assumption, though accepted for decades, is not completely established. There have been some recent temperature measurements by laser scattering indicating wide differences between electron and ion temperatures at the centre of an arc plasma. A further problem in treating the arc column has been the treatment of radiation absorption. Ultra violet radiation emitted at the arc centre, is generally reabsorbed at the edge of the arc.
(b) The anode plasma sheath: Certainly there are non thermodynamic equilibrium effects in the electrode sheath regions. At the anode there is a very large gradient in the electron density from the density in the main arc plasma and the density at the surface of the anode. This density gradient is so large that the diffusion current of the electrons is calculated to be larger than the total current, unless there is a negative anode fall voltage to provide a retarding influence on the electrons.
(c) The cathode plasma sheath: Modeling the plasma sheath for the cathode also involves basic physics considerations which have not been clearly established. Somewhere, either in the sheath or from the cathode, electrons must originate to provide the electrons constituting the flow of current in the main arc column. For thermionic cathodes, such as tungsten and carbon, thermionic emission provides these electrons. But for non- thermionic cathodes, such as is generally the case in arc welding where the cathode is iron or steel, there needs to be a consideration of space charge effects in the cathode sheath.
(d) The electrodes: In general, modeling the electrodes just involves the energy balance equation to calculate electrode temperatures. Nevertheless energy transfer needs to be taken into account when electrons leave the cathode or are absorbed by the anode, and this involves a knowledge of the work function of the metal. If molten liquid is being modeled at the electrodes, as in arc welding, knowledge is needed of the surface tension coefficient of the molten liquid. This surface tension coefficient can change by a factor of two with small impurities of a fraction of 1%.

SUCCESSES IN ARC MODELING

Arc modeling generally consists of solving the conservation equations of mass, energy, momentum and current, for the arc column and also for the arc electrodes. These four equations determine the principal arc quantities of temperature, pressure, plasma velocity and electric potential for any given arc current. The material functions of the plasma, the electrodes and also, for arc welding, the molten electrodes, namely thermal and electrical conductivity, viscosity, density and specific heat, need to be incorporated as a function of temperature. With the availability of modern high speed computers, and the careful use of recently developed numerical methods, this level of detailed computation is quite feasible. Approximations need to be made for treatment of the electrode sheath regions. However for currents of 100A or more, detailed treatment of these regions can be omitted.
(1) Arc lamps: A principal class of arc lamps consists of an arc in mercury vapor plus additives in a cylindrical quartz tube. Then the arc is approximately uniform axially, so that convective effects can be neglected, and for the principal properties of the arc column, electrode effects can also be neglected. Then these column properties can be modeled simply by solving just the energy balance equation for the plasma, without any consideration of the electrodes or plasma convection. Such a solution yields arc temperatures as a function of radius, radiation emission, and column electric field strengths, for any given current and arc tube radius.
(2) Arc welding: Recently calculations have been made appropriate for MIG (Metal Inert Gas) arc welding. These calculations involve a consideration of the conservation equations of mass, energy, momentum and current for the plasma, the electrodes and also the molten region of the electrodes, all in two dimensions. A feature found from MIG welding, is that there is a fairly discreet mode change at a particular current when the size of the molten metal drops from the wire electrode changes from being larger than the welding wire at low current, to smaller than the diameter of the welding wire at high current. Predictions from modeling confirm this mode change and elucidate its mechanism as resulting from the pinch effect of the self magnetic field of the current in the drop.
(3) Lightning: An intriguing aspect of lightning is that before each lightning strike, there is the phenomenon of the "stepped leader". A step of plasma approximately 50m long lights up for ~1ms, and then is dark for ~50ms. Then a second step, also about 50m long lights up from the end of the first step, but the first step is not again illuminated. The second step also becomes dark for about 50ms, and is followed by a third step etc. Branching at a new step can occur. This complicated, but regular, behaviour, can also be explained by modeling. In this case it is the equations of conservation of electrons, negative ions and positive ions that are solved, together with Poisson's equation, to account for space charge distortion of the electric field.

The performance of most plasma generators using electric arcs is determined by the design of the electrodes. While the cathode design is the dominant factor in determining the limitations in torch operating current and power level as well as life between maintenance, the anode design dominates the functional performance for most torches, i.e. the heat and momentum transfer to the materials to be processed. Plasma torch electrodes are conveniently divided into the following categories: (1) thermionic cathode, (2) non-thermionic (cold) cathode, (3) anode with arc axis and flow perpendicular to the surface, and (4) anode with the arc axis and the flow parallel to the surface. All possible combinations are encountered in commercially available torches.

Thermionic cathodes consist of a refractory material in form of a rod or button, frequently with addition of a low workfunction material to reduce the cathode spot temperature. The cathode spot temperature is determined by the arc current density and the electron emission characteristics of the cathode material because the electron emission is the dominant cooling mechanism. Since the low workfunction material has usually a lower evaporation temperature than the cathode spot operating temperature, this material is lost by evaporation and a careful materials balance for the material has to be performed for optimal cathode design. This balance includes material transport by diffusion in the refractory metal matrix, evaporation and redeposition in form of ion flux or in form of regular vapor deposition in the cooler regions of the cathode. For high current densities, the cathode spot will be molten, and the additional effects to be considered are solution of plasma gas atoms in the cathode material and ejection of molten metal droplets from the cathode. Thermionic cathode design also needs to consider the arc diameter in relation to the diameter of the emitting spot. For example, too strong cooling of the cathode by conduction may reduce the size of the emitting spot, leading to higher electric fields in the sheath region which may increase erosion. Plasma torches with thermionic cathodes span the range of less than 100 W to 100 MW and the range of arc currents from about 1 A to 100 kA for operation with non- oxidizing gases.

Cold cathodes usually consist of a water-cooled metal. The electrons are supplied to the arc by evaporation and ionization of the metal in a very small cathode spot. Consequently, electron emission is necessarily associated with materials loss. In comparison with thermionic cathode attachments, the cold cathode attachment is by nature unsteady, since it depends not only on the cathode material and the arcing gas, but also on the physical and chemical surface conditions which change during the arcing process. For minimizing erosion a forced movement of the cathode attachment is used by means of magnetic fields and/or transverse fluid flow. This motion can be enhanced by the addition of impurities to the plasma gas which apparently result in changes of the surface condition which then increase the arc attachment velocity. Cold cathode torches are available for power levels from about 100 W to 10 MW, and for currents from 100 A to about 3 kA.

The anode configuration with the anode surface perpendicular to the arc axis and the plasma flow directed towards the anode is a typical arrangement in transferred arc reactors. Control of the heat transfer to the anode is the design objective. Several factors contribute to the heat flux, in general the condensation of the electron on the cathode material and the transfer of the electron enthalpy being the most important ones. The radial distribution of the anode heat flux is strongly dependent on the flow configuration. For a sufficiently strong flow directed towards the anode surface, i.e. a typical stagnation flow pattern, the plasma covers a wider area of the anode surface than that of the arc cross section, and the strong electron density gradients between the anode surface and the plasma lead to an electron diffusion flux which can carry the current. A negative anode fall is the consequence for this diffuse attachment. If the flow towards the anode is low, a constriction of the arc will take place and an increase of the arc temperature towards a maximum right in front of the anode surface. This constriction results in the formation of an anode jet directed towards the incoming plasma flow and in the formation of a stagnation point some distance away from the anode surface. The heat flux distribution in this latter attachment is much more strongly peaked than in the case of the diffuse attachment, although the total heat transfer may be the same.

For a configuration in which the arc axis is parallel to the anode surface and a flow is superimposed to the arc, the anode attachment is usually unsteady. The attachment forms a current path across a cold gas boundary layer between the anode surface and the arc column. The motion of the anode attachment is determined by the relative magnitude of the drag caused by the flow in the cold boundary layer on the highly viscous plasma channel between the arc column and the anode surface, and of the Lorenz forces due to the self-magnetic field and the curvature of the current path, and this relative magnitude is in turn determined by the thickness of the cold gas boundary layer. For a relatively thick boundary layer, a downstream motion of the arc anode attachment is observed until the potential gradient across the boundary layer at an upstream location becomes sufficiently large for a breakdown resulting in a periodic variation of the arc length (restrike mode). Increase of the arc diameter due to a change in arcing gas for the same arc current and the same anode diameter will reduce the boundary layer thickness and facilitate a breakdown across the boundary layer. A more random variation of the arc length is the consequence (takeover mode). Increasing the arc current for the same channel diameter and the same plasma gas flow rate will also result in a decrease in the boundary layer thickness and a simultaneous increase in the magnetic forces driving the arc attachment upstream. A change in operating mode to a stationary short arc may occur. While the movement of the anode attachment has the advantage of reducing anode erosion, the resulting variation in arc voltage and power will produce variations in the enthalpy levels in the plasma jet and, therefore, in the heating rates, and result in fluid dynamic instabilities of the jet. On the other hand, operation in the stationary attachment mode will result in high anode erosion rates, lower power levels and, because of the short arc, in lower torch efficiencies. Quantitative description of these phenomena requires a three dimensional dynamic model which is presently not yet available.

The specific plasma torch application will dictate the combination of cathode and anode arrangement, and the optimal operating mode for the chosen combination.

INTRODUCTION

Plasma torches with dc electric arcs are used for generation of high temperature and high velocity plasmajets. Extremely high plasma temperatures and velocities can be achieved in torches with arcs stabilized by liquid, where arc column is surrounded by liquid vortex which is created in cylindrical chamber with tangential injection of stabilizing liquid. Plasma is produced by heating and ionization of vapor released from the inner surface of the vortex due to interaction with the arc. As thermal loading of the liquid wall surrounding the arc can be substantilly higher than the loading of walls of arc chambers in common gas stabilized torhes, high power arcs can be stabilized at very 1ow mass flow rates of vapor and thus extreme plasma enthalpies can be achieved. The mass flow rate in liquid stabilized torches can not be controlled independently. It is determined by evaporation rate of the liquid wall which is determined by the balance of energy transfer from the conducting arc column to the liquid wall. The paper describes the mechanisms of the liquid stabilized arcs on the basis of theoretical model and presents the survey of experimentally determined properties of plasma torches with water and mixtures of water with ethanol.

MECHANISMS OF LIQUID STABILIZED ARCS AND THEIR MODELING

Relations between parameters of generated plasmajet, material properties of the liquid and its vapor and geometry of stabilizing chamber can be determined from theoretical models of the liquid stabilized arc. The model used for description of liquid stabilized arcs is based on the solution of equations describing radial and axial transfer of energy and mass in the stabilizing chamber. The volume of the chamber can be divided into four radial zones. conducting arc core, sheath of vapor between arc and water surface, boiling layer at the inner surface of the liquid vortex arid water vortex itself. The energy and mass transfer mechanisms between these four radial zones control all arc characteristics and properties of generated plasma jet. The relative importance of various energy transfer mechanisms in the arc column was determined on the basis of the model. Due to extremely high temperatures and relatively low mass flow rates, radiation transfer is very important. The model describes the radiation of plasma in arc core where very high radial gradients of temperature exist as well as an absorption of radiated, power in the sheath of vapor created between the conducting column and the wall and absorption of radiation in the layer of boiling Iiquid, As exact solution of the problem is not possible, simple empirical model was formulated based on net emission coefficients and factors expressing fractions of total arc power absorbed in the separate regions, surrounding the arc column. The empirical fraction factors were determined from the experimerits: The solution of the model resulted in a system of curves representing scaling relations and effect of material properties for liquid stabilized arcs. In the computations the equilibrium transport and thermodynamic coefficients of liquid and its vapor were used.

PROPERTIES OF LIQUID STABILIZED TORCHES

Experiments were performed with arc stabilized by water and mixtures of water with ethanol. Measurements of arc characteristics were performed together with ineasurements of power balances of al parts of the system and with diagnostics of generated plasma jet. Very high plasma temperatures, enthalpies-and plasma flow velocities were found in the experiments. The measured mass flow rates of plasma were almost one order lower than flow rates in common gas stabilized torches. Effect of liquid properties on characteristics of arc and properties of plasma was studied. Addition of small amount of ethanol into water lead to substantial increase of arc power and, of course, to change of plasma composition. Despite of the higher arc power, the plasma temperature is reduced by addition of ethanol. This is the consequence of higher evaporation rate of liquid wall surrounding the arc column as well as the consequence of change of material properties of arc plasma. Lower boiling temperature of ethanol together with lower latent heat of evaporation lead to higher evaporation rate and reduction of power loss to the flowing liquid surrounding the arc column. Addition of ethanol thus causes increase of the power efficiency of the torch.

The performance characteristics of liquid torches in plasma processing applications result from very high efficiency of utilization of plasma energy for treatment of substances injected into plasma. The effect of plasma jet parameters on operation characteristics in plasma processing can be illustrated by curves representing effect of loading on heat and momentum transfer to the powder in plasma spraying. Due to injection of powder the temperature and velocity of plasma are reduced. Thus, the heat and momentum transfer to the particles is dependent on amount of treated material. The curves representing these dependencies were calculated from measured parameters of water torch and from published data on gas torches. The relation between heat flux potential representing conditions around particles and fraction of total heat flux in the jet spent for powder heating was determined. It is shown that substantially higher part of jet power can be spent for particle heating in water torch than in common gas torches. It is the cause of extremely high powder throughputs reported for water plasma torches.

Presence of oxygen in water vapor plasma causes very high erosion rate of electrodes, especially due to production of fragile oxides on the electrode surface. Thus; special electrode materials and special configurations are used in torches with water. Consumable graphite cathode can be used, or for lower currents hafnium and zirconium cathodes were tested. Anodes are created by rotating water cooled disc which is located outside the arc chamber several mm downstream of the exit nozzle. Thus, part of the plasmajet between nozzle exit and point of anode attachment with length equal to two or three diameters of the nozzle is heated by passage of electric current. This arrangement leads to some special characteristics of plasina jet which originate in Joule heating of the jet as well as in interaction of secondary anode jet with the main jet.

REFERENCES

• Hrabovsky M , Konrad M.; Kopecky V.; Sember V., Processes and properties of electric arc stabilized by water vortex, IEEE Trans on Plasma Science 25 (1997), No. 5, pp. 833-839.
• Hrabovsky M., Water stabilized plasma generators, Pure & Applied Chemistry 70 (1998), No, 6, pp. 1157 - 1162.

A two-temperature variable-density, treatment has been developed for description of high current free burning arcs[1]. The treatment includes the arc and the electrodes and considers departures from local thermodynamic equilibrium (LTE) and local chemical equilibrium (LCE) in the plasma. For a 200 A arc in pure argon at 1 atm, we calculate large differences between the temperatures of the electrons and the heavy particles only in the plasma regions near the cathode tip and the outer parts of the arc. However, we predict large departures from LCE throughout the plasma, due to overpopulation of the ground state level of the neutral atoms caused by the injection into the arc core of a large mass flow of cold gas, due to arc constriction at the tip of the cathode.

The plasma and the electrodes are described using temperatures T_e and T_h, pressure P, velocities v_r and v_z, current densities j_r and j_z, electric potential V and densities n_e, n₀ and n_i. Subscripts e, i, 0 and h refer to electrons, ions, atoms and heavy particles respectively. We consider a system with neutral atoms, electrons and positive ions. We assume that the plasma is in partial LTE (PLTE), and that the plasma kinetics is dominated by impact ionisation and three body recombination. The equations describing the arc and the electrodes are the mass continuity equation, the energy equations for the electrons and heavy particles, the momentum equation, the current continuity equation, Ohm's law and one of Maxwell equations. We also solve the electron continuity equation together with Dalton's law and the charge neutrality condition to determine the plasma composition throughout the arc.

The material functions of the plasma, including electrical conductivity s, electron thermal conductivity k_e, heavy particle thermal conductivity k_h and viscosity m are calculated using a modified Devoto's model[2]. We find that the effect of departures from LTE on s, k _e and k_h is mostly due to variation of the number densities of the electrons and the heavy particles. However, for the viscosity, we find that both differences between n_e and n_e^LTE, and differences between T_e and T_h can have a large effect on the calculated values m.

For temperatures less than 5000~K, we assume that the plasma is in LTE, and there are no electrode sheath regions included in the present model. Input parameters for the model are the material functions of the electrodes, the net radiation loss from the plasma, and the arc current, and the output includes a two-dimensional distribution of temperature, velocity, pressure, electric potential and electron number density.

Results are presented for an arc current of 200~A, an arc length of 5~mm, a gas flow of 5 l/min of pure argon and a ThW cathode of 3.2 mm diameter and 60^o included cone angle. The calculations show that for the plasma region in front of the cathode tip, there are small differences between the temperatures of the electrons and the heavy particles, in agreement with previous calculations in the literature[3]. For the plasma region immediately surrounding the cathode, we calculate differences of up to several thousand degrees. The variation of the plasma emission coefficient at Ar I lines, that is observed experimentally near the cathode tip for arcs in argon at axial positions between the cathode tip z=0 and z=3 mm in the plasma, is calculated to occur over large parts of the arc and is due to the effects of the plasma flow. Also the results highlight the strong effects of the arc-cathode interactions on the plasma conditions, due to the important effects of the current distribution at the surface of the cathode on the arc characteristics.

1. J. Haidar, J. Phys. D: Appl. Phys. 32, 263 (1999).
2. R. S. Devoto, Phys. Fluids 16, 616 (1973).
3. K. C. Hsu and E. Pfender, J.Appl.Phys. 54, 4359 (1983).

   ---

   EROSION OF THE COLD ELECTRODE AS AN ABLATION PROCESS IN UNSTEADY ARC SPOTS

   A. Marotta†, L. I. Sharakhovsky†‡ and A. M. Essiptchouk†‡

   †Instituto de Física "Gleb Wataghin" Universidade Estadual de Campinas, Unicamp, 13083-970, Campinas, São Paulo, Brazil
   ‡Permanent address: The Luikov Heat & Mass Transfer Institute, P. Brovki str., 15, 220072, Minsk, Rep. Belarus

   A simple macroscopic model is presented for the erosion of copper electrode in unsteady arc spots, based on consideration of erosion as a heat ablation process of the electrode material. Experimental results on Electric Arc Heaters and Electrical Discharge Machining equipment, taken from our and other authors' experiments, have shown a sufficiently good agreement with the model.

   INTRODUCTION

   The problem of the erosion of copper electrodes is the most actual for the technological electric arc heaters (EAHs). Because of extremely high heat fluxes, these electrodes can be used only during the high-speed displacement of the arc spots. It is known, that, depending on the properties of the electrode surface, the arc spot in EAHs can move, either continuously or jumping-like. The macroscopic model for the particular case of the continuous motion of the spot was presented earlier1. Here, we are developing further a more general approach for both aforesaid regimes of displacement of the arc spot, the continuous one and the jumping-like (or step-wise regime).

   THEORETICAL MODEL

   We consider the erosion of the electrode as a process of ablation of the electrode material under the effect of high intensity heat fluxes in the arc spot. This process we characterize with some value of the effective enthalpy of erosion h_ef. For our model we will apply the following main assumptions: (1) The Joule heating of the copper cathode immediately beneath the arc spot can be neglected and the arc spot can be considered as a surface heat source²; (2) The state of motion of the arc spot satisfies the conditions for the Fourier number 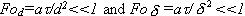, where t is the time of heating the electrode within the arc spot, d is the diameter of the arc spot, d is the thickness of the electrode wall and a is the thermal diffusivity of the electrode material; (3) During the period the heating of the electrode within the arc spot occurs under the boundary conditions of the second kind³ with the prescribed constant heat-flux density q₀ = jU, where j is the current density in the arc spot, U is the volt-equivalent of the heat flux in the arc spot, which is equal to the ratio of this flux to the current and t₀ is the time of the heating of the electrode material to the fusion temperature .; (4) During the period the temperature of the electrode surface is constant and equal to the fusion temperature , which corresponds to the boundary conditions of the first kind³; (5) During the step-wise motion, the arc spot exists only at fixed discrete positions with infinitely short time of displacement from one point to another. For we take as the initial condition the temperature field, obtained from the solution of the preceding problem, i.e., at (see assumption (3)).

   We consider erosion as the result of the inbalance between the heat supply by the arc spot and heat removal within the arc spot during the period . As a result, certain part of the supplied heat is spent on the ablation of the electrode. We have obtained the following simple relationships for the specific erosion of the cold electrode in unsteady arc spots (in kg/C):

   

   Here g₀ is some constant value (approximately 2-3 mg/C); b, w₁ and w₂ are functions of the dimensionless parameter (not shown here); n is the velocity of the arc; l is the thermal conductivity of the electrode; T is the surface temperature of the electrode; I is the current; n=L/d; L is the step length of the arc for the step-wise motion. The domains of definition of W and are correlated as following: W=0 for _>1 and 0< W <1 for < 1. For L=d and for the continuous model n=1.

   COMPARISON OF THEORY AND EXPERIMENT

   In Fig. 1a we show the results of generalizing our experiments on the study of erosion of copper cathode of EAH in the form of eq. (1) with both models, the continuous one and the step-wise motion. During these experiments we measured the main parameters included in the dimensionless parameter f (see eq. 4), i.e., the current I, the arc velocity nv and the surface temperature of the electrode T. In Fig. 1a we visualize with black the points, obtained with the constant values of the magnetic field, water-cooling and plasma-forming gas mass flow rates. The remaining points are obtained for random variation of all parameters within the ranges: current 24-1000 A, arc velocity 19-344 m/s, temperature of cathode 300-1073 K. One can see, that both models give close results, but the step-wise model gives somewhat higher coefficient of correlation in comparison with the continuous one (0.95 and 0.942, respectively). Processing of our experiments with the continuous and the step-wise models give some different values of effective enthalpy of erosion – 66 and 81 MJ/kg and microerosion – 3.1 and 2.4 mg/C, respectively (see Fig.1a). In Fig. 1b we give a qualitative comparison (qualitative, because the authors did not measure the temperature of the cathode) for the continuous model with the Szente's et. al. experiments on the investigation of the influence of the arc velocity on the erosion⁴. The temperature regime of the experiment was evaluated by matching the theoretical curve with the experimental point in the transition region of the descending branch of the curve to the horizontal one. As is evident, being adjusted to one single point, the model qualitatively reproduces well the arrangement of the remaining experimental points. In Fig. 1b we obtained for the effective enthalpy of erosion a value higher than for our experiments – about 130 MJ/kg. The authors⁴ utilized pure electrolytic copper as the cathode and argon-nitrogen mixtures as the plasma-forming medium. We utilized in our experiments commercial copper and nondried air from the technical manifold. In Fig. 2c we give a qualitative comparison of the step-wise model with experiments obtained on electrical discharge machining⁵ (EDM) equipment. Here, the authors have used steel as the electrode, and accurately adjustable pulse duration, taken as the coordinate x. Ìn this case we have chosen the value of the current density as 2*10⁶ A/cm² from the condition of agreement of the first of the lower point with the theoretical curve. With an effective enthalpy of 4.8 MJ/kg we obtained a good agreement between the theoretical curve and the experimental data (see Fig. 2b).

   CONCLUSION

   We obtained a sufficiently good agreement between theory and experiments on EAHs and EDM equipments. As a result, we obtained the important parameters for the calculation of erosion, the effective enthalpy of erosion h_ef and the specific microerosion g₀. For copper cathode in air they are equal, approximately to 70-130 MJ/kg and 2-3 mg/C, respectively, depending on the type of the copper material and the plasma chemical composition. For steel in the EDM process, the effective enthalpy is equal to about 5 MJ/kg. This demonstrates the presence of a considerably stronger mechanism of erosion in EDM equipment than in EAHs, which was to be expected.

   We thank Mr. A. A. B. do Prado and Mr. J. B. Pinheiro for their technical assistance in this work. We acknowledge the financial support of CNPq, FAPESP and FINEP of Brazil.

   REFERENCES

   • A. Marotta and L. I. Sharakhovsky, "A theoretical and experimental investigation of copper electrode erosion in electric arc heaters. I: The thermophysical model," J. of Phys. D: Appl. Phys., vol. 29, pp. 2395-2403, 1996.
   • A. V. Luikov, A. E. Borovchenko, V. I. Krylovich, V. V. Toropov, L. I. Sharakhovsky, and A. S. Shaboltas, Heat Transfer in Near-electrode Region of the Electric Arc Blown, Trans. of Int. Symp. JSME, Tokyo, pp 113-119, 1967.
   • A. V. Luikov, Analytical Heat Diffusion Theory, Academic Press, New York and London, 1968.
   • R. N. Szente, R. J. Munz, and M. G. Drouet, "Effect of the arc velocity on the cathode erosion rate in argon nitrogen mixtures" J. of Phys. D: Appl. Phys. Vol. 20, pp. 754-756, 1987.
   • D. D. DiBitonto, P. T. Eubank, M. R. Patel, and M. A. Barruffet, "Theoretical models of the electrical discharge machining process. I. A simple cathode erosion model," J. Appl. Phys. Vol. 66, no. 9, pp. 4095-4103, 1989.